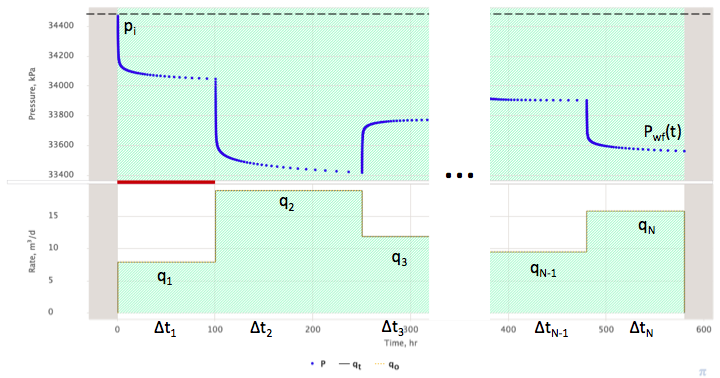
Multi-rate drawdown test
| Fig. 1. Multi-rate drawdown test |
Given:
- a discrete flowrate history
\{ \, (\Delta t_1, q_1) , \, (\Delta t_2, q_2) , \, (\Delta t_3, q_3) , \, (\Delta t_{N-1}, q_{N-1}) , \, (\Delta t_N, q_N) , \, \} (see Fig. 1)
- vertical well and homogeneous reservoir with no boundaries
- duration of the last drawdown interval \Delta t_N reaching radial flow regime
the superposition time t_s is defined as:
| (1) | \ln t_s = \ln (t-t_{N-1}) - \sum_{i=1}^{N-1} \frac{q_i - q_{i-1}}{q_{N-1}} \ln (t-t_{i-1}) = \ln \Delta t - \sum_{i=1}^{N-1} \frac{q_i - q_{i-1}}{q_{N-1}} \ln \left( \Delta t + \sum_{i=1}^{N-i} \Delta t_i \right) |
The pressure response at time moment t=t_N will be given by formula:
| (2) | p_{wf}(t_s) = p_i + \frac{q_N B}{4 \pi \sigma} \ \ln t_s |
which has the same format as for a single rate drawdown test with flowrate q_N, and duration t_s and can be interpreted using Type-Curve Matching.
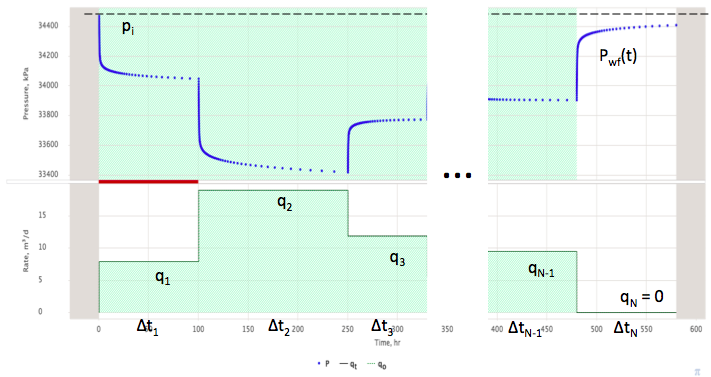
Multi-rate build-up test
| Fig. 2. Multi-rate build-up test |
Given:
- a discrete flowrate history
\{ \, (\Delta t_1, q_1) , \, (\Delta t_2, q_2) , \, (\Delta t_3, q_3) , \, (\Delta t_{N-1}, q_{N-1}) \, \} followed by a shut-in period
(\Delta t_N, q_N = 0) (see Fig. 2)
- vertical well and homogeneous reservoir with no boundaries
- duration of the last shut-in \Delta t_N reaching radial flow regime
the superposition time t_s is defined as:
| (3) | \ln t_s = \ln (t-t_{N-1}) - \sum_{i=1}^{N-1} \frac{q_i - q_{i-1}}{q_{N-1}} \ln (t-t_{i-1}) = \ln \Delta t - \sum_{i=1}^{N-1} \frac{q_i - q_{i-1}}{q_{N-1}} \ln \left( \Delta t + \sum_{i=1}^{N-i} \Delta t_i \right) |
The pressure response at time moment t=t_N will be given by formula:
| (4) | p_{wf}(t_s) = p_i + \frac{q_{N-1} B}{4 \pi \sigma} \ \ln t_s |
which has the same format as for a single rate drawdown test with flowrate q_{N-1}, and duration t_s and can be interpreted using Type-Curve Matching.
See Also
Petroleum Industry / Upstream / Subsurface E&P Disciplines / Well Testing / Pressure Testing / Pressure Transient Analysis (PTA) / PTA Diagnostic Plot
[ Well & Reservoir Surveillance ] [ Pressure Diffusion ] [ Pressure log-log plot ]