Two different functions of real argument $\begin{array}{l}x \in \mathbb{R}\end{array}$ are called this way:

(1)	$\begin{array}{l}\displaystyle {\rm Ei}(x) = - \int_{-x}^{\infty} \frac{e^{-\xi}}{\xi} \, d\xi\end{array}$

(2)	$\begin{array}{l}\displaystyle {\rm E_1}(x) = \int_{x}^{\infty} \frac{e^{-\xi}}{\xi} \, d\xi\end{array}$

which are related to each other as:

(3)	$\begin{array}{l}\displaystyle {\rm Ei}(x) = - E_1(-x)\end{array}$

There is a trend to moving from $\begin{array}{l}\rm Ei\end{array}$ definition (which was dominating in the past) towards $\begin{array}{l}\rm E_1\end{array}$ which becomes more and more popular nowdays.

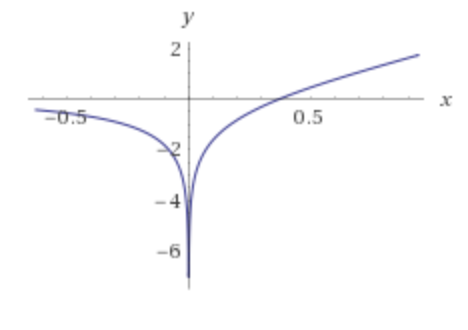
Fig. 1. A sample graph of $\begin{array}{l}y ={\rm Ei}(x)\end{array}$

Approximations

$\begin{array}{l}|x| \ll 1\end{array}$

$\begin{array}{l}|x| \gg 1\end{array}$

(4)	$\begin{array}{l}\displaystyle {\rm Ei}(x) = \gamma + \ln \|x\| + \sum_{k=1}^\infty \frac{x^k}{k\cdot k!}\end{array}$

(5)	$\begin{array}{l}\displaystyle {\rm Ei}(x) = e^x \, \left[ \frac{1}{x} + \sum_{k=2}^\infty \frac{(k-1)!}{x^k} \right]\end{array}$

where $\begin{array}{l}\gamma = 0.57721\end{array}$ ... is Euler–Mascheroni constant

$\begin{array}{l}-1 \ll -x < 0\end{array}$

$\begin{array}{l}0 < x \ll 1\end{array}$

(6)	$\begin{array}{l}\displaystyle {\rm Ei}(-x) \sim \gamma + \ln x\end{array}$

(7)	$\begin{array}{l}\displaystyle {\rm Ei}(x) \sim \gamma + \ln x\end{array}$

Application

The $\begin{array}{l}\rm Ei\end{array}$ -based function:

(8)	$\begin{array}{l}\displaystyle {\rm w}(t, r) = E_1 \left( \frac{r^2}{4 \, t} \right) = -{\rm Ei} \left( - \frac{r^2}{4 \, t} \right)\end{array}$

defines a solution planar axial-symmetric diffusion equation:

(9)	$\begin{array}{l}\displaystyle \frac{\partial {\rm w}}{\partial t} = \frac{\partial {\rm w}^2}{\partial^2 r} + \frac{1}{r} \frac{\partial {\rm w}}{\partial r}\end{array}$

(10)	$\begin{array}{l}\displaystyle {\rm w}(t=0, r) = 0\end{array}$

(11)	$\begin{array}{l}\displaystyle {\rm w}(t, r=\infty) = 0\end{array}$

(12)	$\begin{array}{l}\displaystyle 0 < = {\rm w}(t, r) < \infty \, , \ \forall (t,r) \in D = \{ t \geq 0, r>0 \} \subset \mathbb{R}\end{array}$

and is widely used in radial heat-mass transfer simulations.

References

https://www.wolframalpha.com/input/?i=Ei(x)

Page tree

Exponential Integral (Ei)

Approximations

Application

References